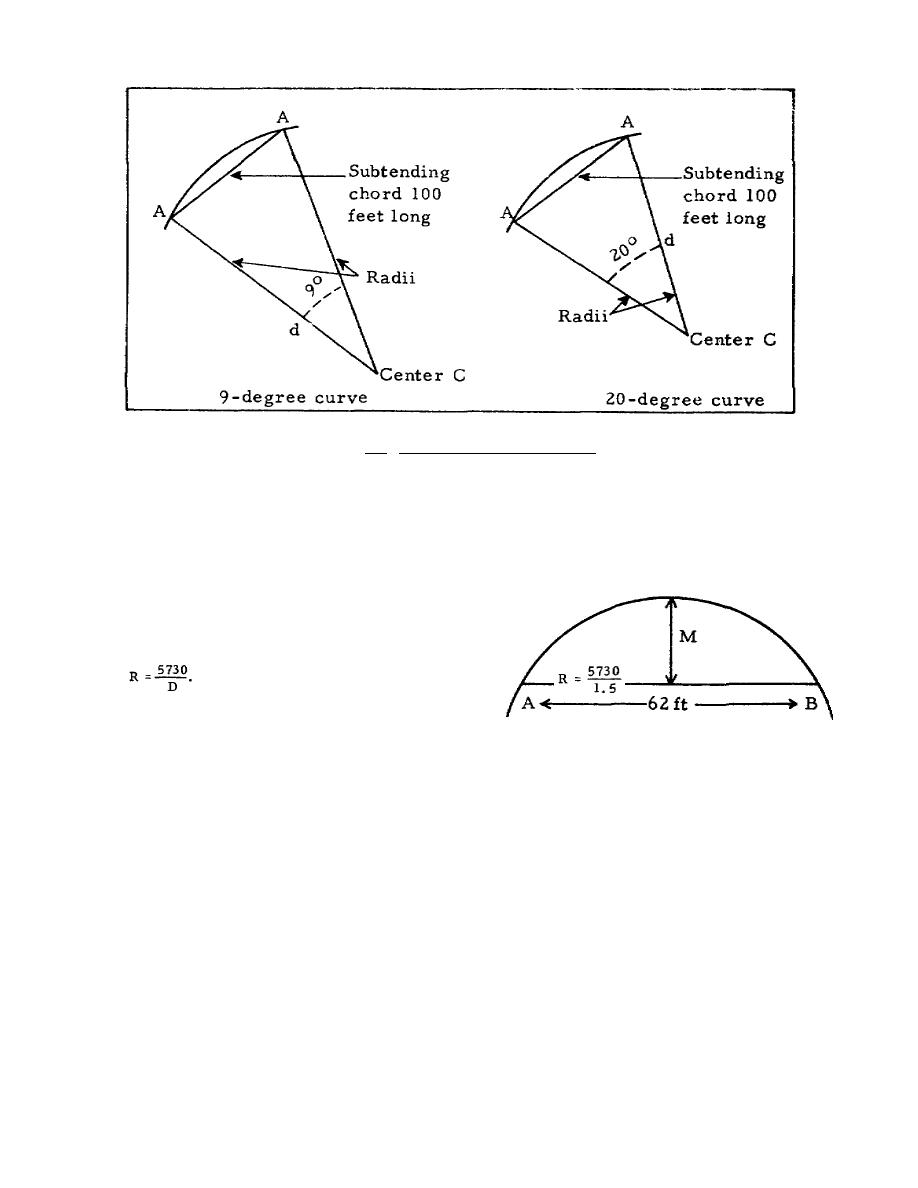
Figure 3.2. Determining Track Curvature.
You can also quickly determine the approximate degree of curvature with a standard 62-foot stringline
cord. This is accurate enough for formula computations of superelevation, discussed in section II. Stretch the
62-foot cord between two points on the inner side of the outside rail--the same method used for the 100-foot
chord in the preceding paragraph and in figure 3.2. The distance measured at the halfway point of the stringline
cord, the 31-foot mark, to the head of the rail in inches is equal to the degree of curvature. The sketch to the right
shows how the measurement is made.
Often, you may need to know the radius of a curve even though
you
already know the degree of curvature. If the curve is not too sharp,
an
approximate value of the radius can be determined by the
following formula in which R represents the radius in feet, D
represents the degree of curvature, and 5730 is a constant.
Thus:
For example, a curve of 1.5 degrees has a
radius of
or 3,820 feet.
3.6.
SUMMARY
The three types of curves used to change the direction of a rail line are the simple or circular, the
compound, and the reverse. They increase the amount of resistance to the pulling power of
65


 Previous Page
Previous Page
