The first six paragraphs of thus section cover the basis of stringlining, the equipment the fieldwork
requires, the marking off of stations, the measuring of ordinates, the evaluation of the figures, and the
calculations involved. The next paragraph discusses the marking of the ends of tangents, spirals, etc. The last
two paragraphs discuss some problems you may encounter in stringlining.
3.17.
BASIS
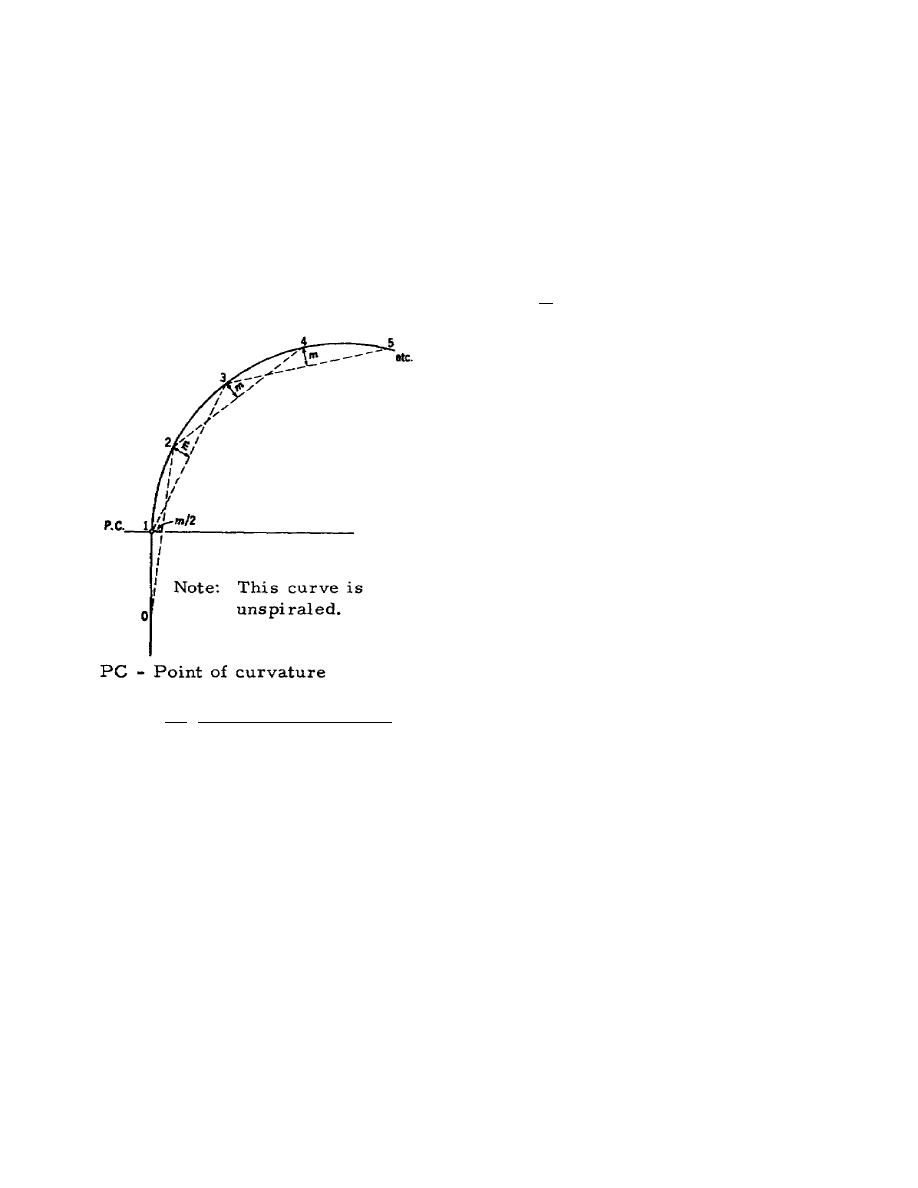
When a string is stretched tautly between two points on the inside or gage side of the outside rail of a
curve, the distance between the midpoint of the string and a point five-eighths of an inch from the very top of the
rail is proportional to the degree of curvature. This is the basis of stringlining. A longer distance between the
midpoint and the rail signifies a sharper curve than does a shorter one. This distance is known as an ordinate,
though some railroaders call it an offset. The measurements marked m in figure 3.4 are ordinates.
When the string used is 62 feet long, the
ordinate in inches equals the degree of curvature.
For example, if a 62-foot string is stretched between
two points on the inside of a rail on the simple
portion of a curve and the ordinate measures 2
inches, then it is a 2-degree curve. If the ordinate
were 3 1/2 inches, it would be a curve of 3 degrees
30 minutes. For this reason, a 62-foot string is
usually used in stringlining.
On a smooth or properly lined simple curve, the
degree of curvature is the same at all points. Since
measured ordinates are equal to curvature, all those
in such a curve should be of equal length. If they
are not, the curve is out of line. Similarly, on a
properly lined spiral, curvature changes at an even
rate. Therefore, ordinates on a spiral measured at
equal distances should vary an even amount from
one to the next.
Figure 3.4. Ordinates on a Simple Curve.
74


 Previous Page
Previous Page
